Structural Optimization Analysis with ANSYS Mechanical16 August 2022

 

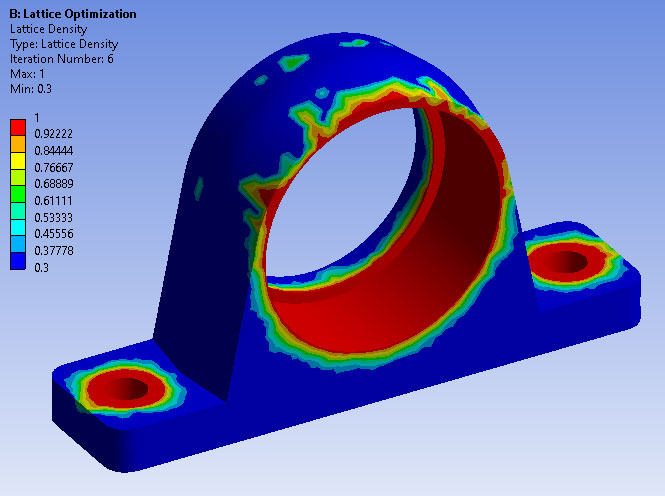
The term structural optimization is commonly used for the optimization of engineering structures for improved strength or stiffness properties and reduced weight or cost.
ANSYS Mechanical 2022R1 provides the following optimization methods:

1. Topology Optimization
There are two options implemented in ANSYS Mechanical software for topology optimization:

• Density Based Optimization: This method performs optimization based on the density of each element of your model. It employs Solid Isotropic Material with Penalization (SIMP) wherein density is forced to approach 0 or 1 rather than varying continuously.
• Level Set Based Optimization: This method directly deals with the boundary of the shape. This enables ANSYS Mechanical to deliver an unambiguous solution.

2. Lattice Optimization

This method enables you to compute an optimal variable density lattice distribution for your geometry.

3. Shape Optimization
This method enables you to optimize the shape of your model by morphing the mesh. As opposed to topology optimization, no topological change is allowed.
We have chosen a bearing house geometry for which we will explain how to perform its optimization in ANSYS Mechanical, step by step.

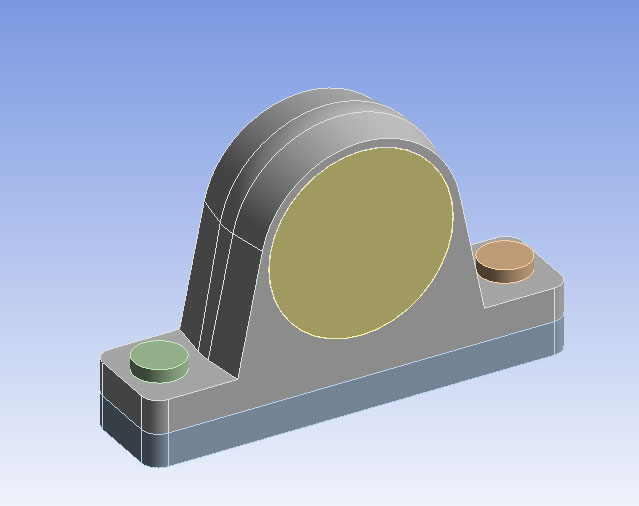
A bearing house is mounted on a plate by two bolts. The bearing house is loaded by a generic bearing (modelled as a shaft) and the plate is fixed at the lower area.

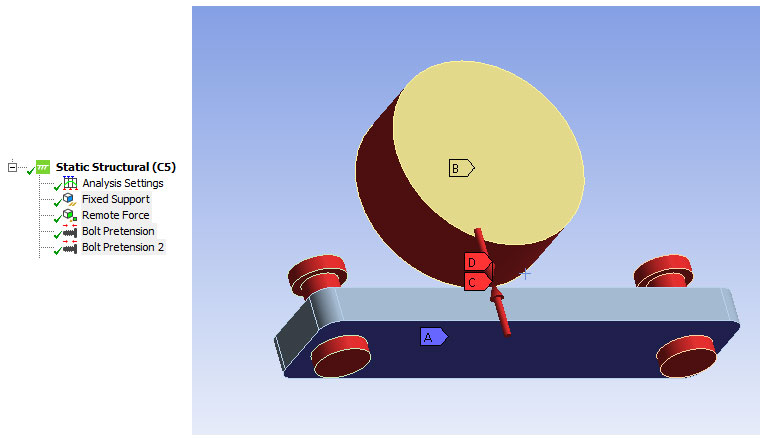
The analysis is done in three steps:
1. The bolts are pretensioned and in the next steps the bolts are locked.
2. Axial and lateral loads on the shaft - case 1 of loads.
3. Axial and lateral loads on the shaft - case 2 of loads.
So, we have two load cases for the bearing/shaft. When there are pretensioned bolts in an assembly, it is always recommended to apply the pretension in the first step prior to any other load and then to lock the bolts.
All contacts are defined as frictional.

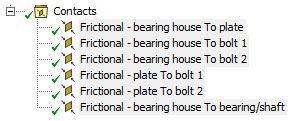
ANSYS Mechanical provides the possibility to do the optimization using the full model (by picking just the desired component for optimization), but with some limitations. The non-linear contacts are not supported yet. We still can do it! In this case, the solution consists in a separate analysis of the desired component for optimization by imposing some equivalent boundary conditions.

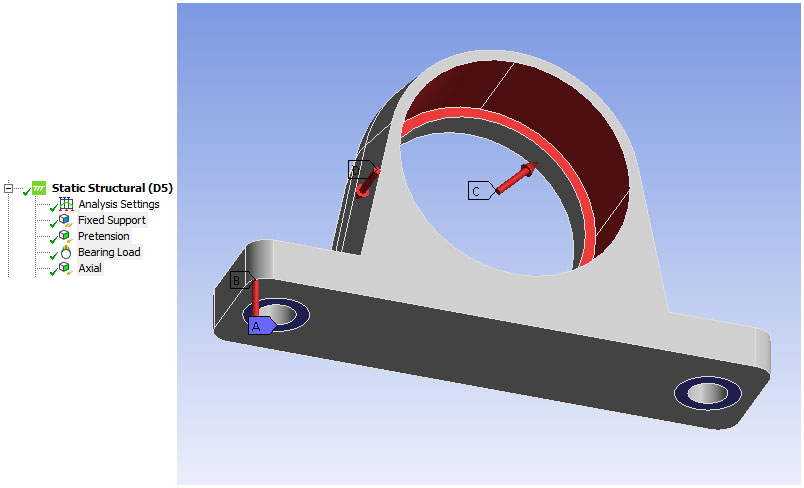
The isolated bearing house model with equivalent boundary conditions can be validated by comparing the equivalent stress maps from the two models for the same load case and with the same contour values.

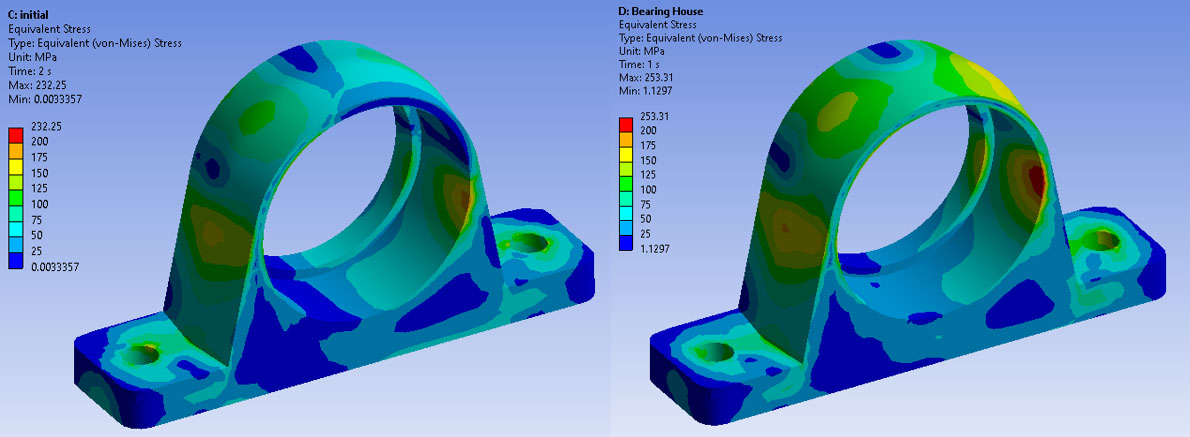
The equivalent stress maps from the assembly model (left) and from the isolated bearing house model (right) are compared. A perfect match is not possible as the boundary conditions for the isolated model are equivalent. Nevertheless, the two maps are close enough and we can proceed with the optimization process. Anyway, the optimized shape is to be inserted in the assembly for the final check.
When the static structural analysis of the isolated bearing house is ready, we continue with the structural optimization, by adding a new simulation block.

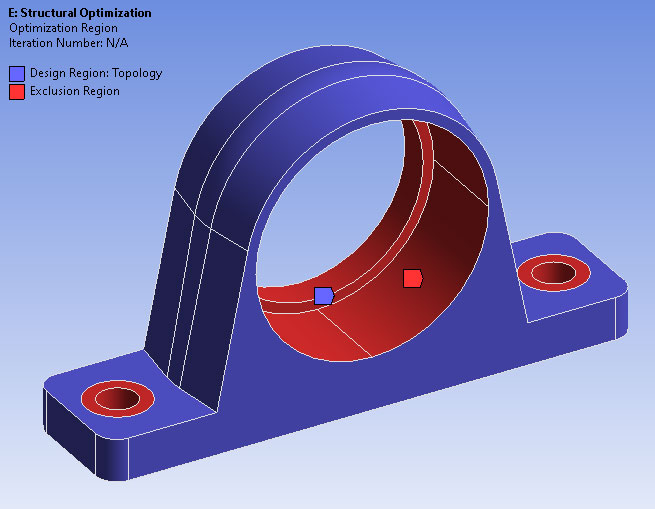
First, we must define the Exclusion Region. Usually, the exclusion region consists of boundary conditions, but other regions can be added (technological issues can be imposed).

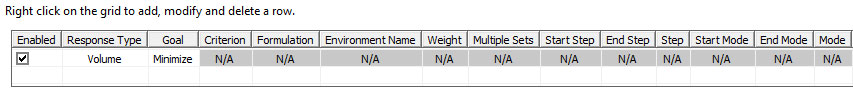
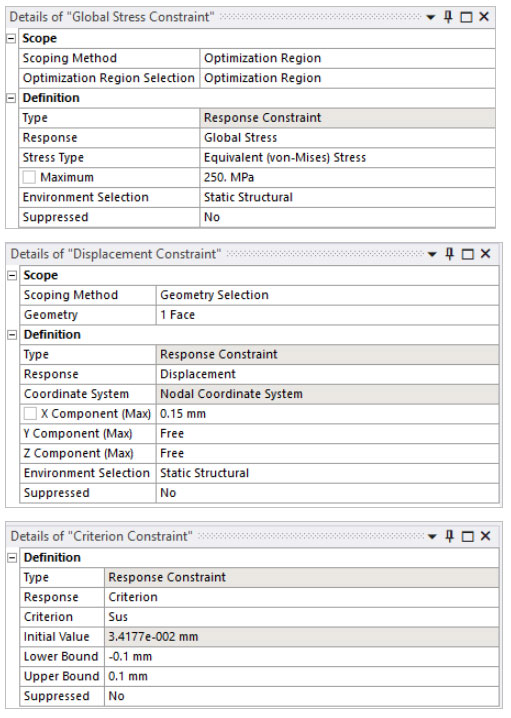
Then, the objective function and different constraints can be defined. In this case the minimization of the volume is the objective function, the global equivalent stress is limited at 250 MPa and displacements in some points are also restricted to some values.

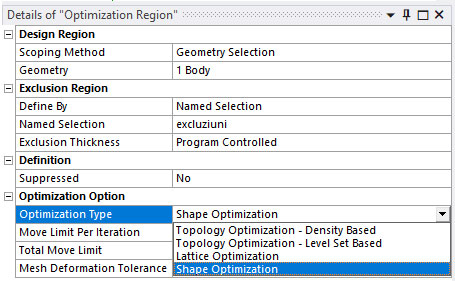
Depending of the type of optimization, we select the desired one.

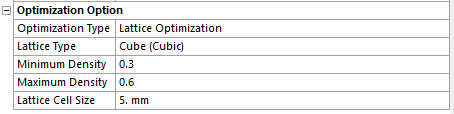
For the lattice optimization there are some particular parameters to be set by the software user.

When all the project runs are done, we obtain the following results, for the same considered initial geometry, but using different methods that are implemented in ANSYS Mechanical:

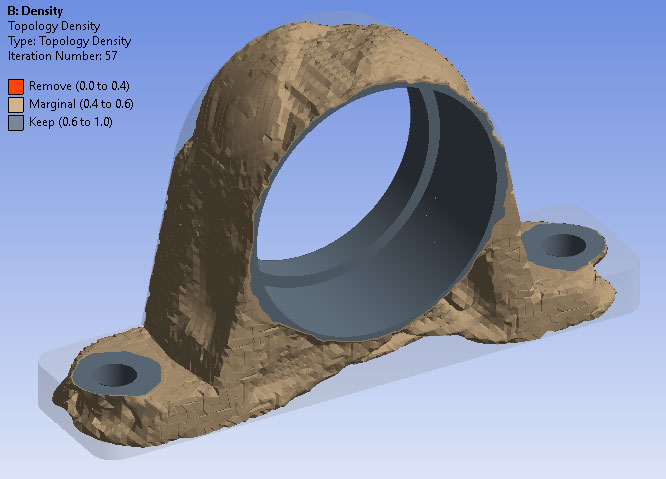
Topology Density method - 63% reduction of the original mass.

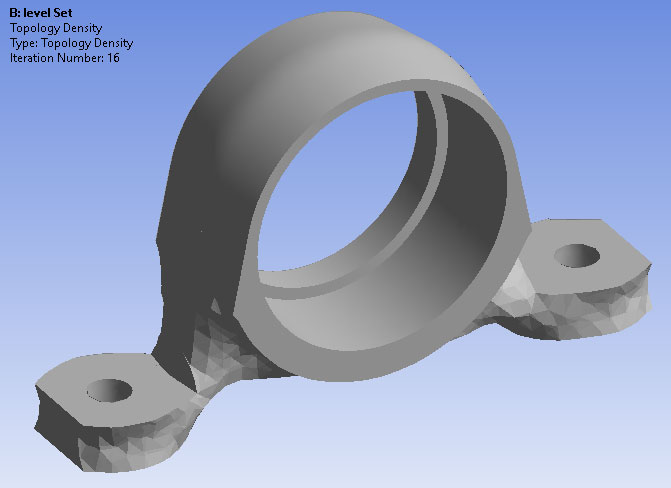
Level Set method - 68% reduction of the original mass.

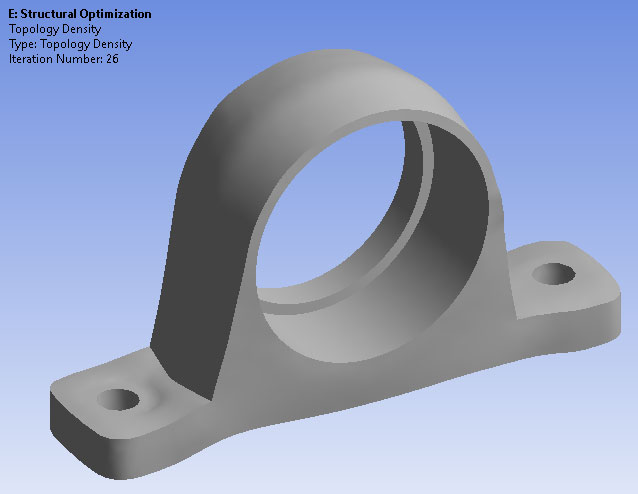
Shape Optimization method - 72% reduction of the original mass.

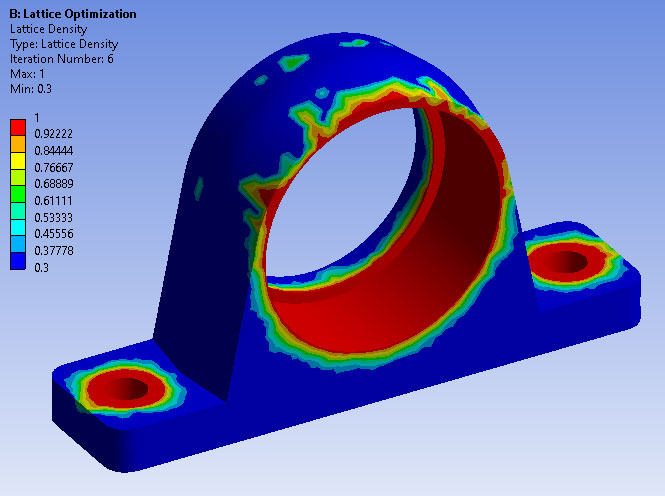
Lattice Optimization method - 41% reduction of the original mass. This method can be applied only for additive manufacturing. Lattices can be visualized when processing further the results with ANSYS SpaceClaim.

Conclusions

 

• Ansys provides very strong tools to perform structural optimization;
• Topology Density Method is ideal to find a starting shape for the structure especially when there are multiple load cases;
• The methods provided by ANSYS Mechanical can be combined, thus using the best of each. For example, after the Level Set optimization is performed, the obtained geometry can be refined even more by using Shape Optimization (which generally applies to components close to optimal shape, as mesh morphology is limited);
• Lattice Optimization is very efficient, but it is limited to 3D Printing;
• There is some computational effort to perform optimization for a structure, but in case of mass production or when low mass is a must (aerospace industry), for sure the gain is worth it.